GridGenerator Namespace Reference

Namespaces
	Airfoil

Creating meshes for basic geometries
template<int dim, int spacedim>
void	hyper_cube (Triangulation< dim, spacedim > &tria, const double left=0., const double right=1., const bool colorize=false)
template<int dim>
void	simplex (Triangulation< dim, dim > &tria, const std::vector< Point< dim >> &vertices)
template<int dim, int spacedim>
void	subdivided_hyper_cube (Triangulation< dim, spacedim > &tria, const unsigned int repetitions, const double left=0., const double right=1., const bool colorize=false)
template<int dim, int spacedim>
void	hyper_rectangle (Triangulation< dim, spacedim > &tria, const Point< dim > &p1, const Point< dim > &p2, const bool colorize=false)
template<int dim, int spacedim>
void	subdivided_hyper_rectangle (Triangulation< dim, spacedim > &tria, const std::vector< unsigned int > &repetitions, const Point< dim > &p1, const Point< dim > &p2, const bool colorize=false)
template<int dim>
void	subdivided_hyper_rectangle (Triangulation< dim > &tria, const std::vector< std::vector< double >> &step_sizes, const Point< dim > &p_1, const Point< dim > &p_2, const bool colorize=false)
template<int dim>
void	subdivided_hyper_rectangle (Triangulation< dim > &tria, const std::vector< std::vector< double >> &spacing, const Point< dim > &p, const Table< dim, types::material_id > &material_id, const bool colorize=false)
template<int dim, int spacedim>
void	cheese (Triangulation< dim, spacedim > &tria, const std::vector< unsigned int > &holes)
	Rectangular domain with rectangular pattern of holes. More...
template<int dim>
void	plate_with_a_hole (Triangulation< dim > &tria, const double inner_radius=0.4, const double outer_radius=1., const double pad_bottom=2., const double pad_top=2., const double pad_left=1., const double pad_right=1., const Point< dim > center=Point< dim >(), const types::manifold_id polar_manifold_id=0, const types::manifold_id tfi_manifold_id=1, const double L=1., const unsigned int n_slices=2, const bool colorize=false)
	Rectangular plate with an (offset) cylindrical hole. More...
template<int dim>
void	channel_with_cylinder (Triangulation< dim > &tria, const double shell_region_width=0.03, const unsigned int n_shells=2, const double skewness=2.0, const bool colorize=false)
template<int dim, int spacedim>
void	general_cell (Triangulation< dim, spacedim > &tria, const std::vector< Point< spacedim >> &vertices, const bool colorize=false)
template<int dim>
void	parallelogram (Triangulation< dim > &tria, const Point< dim >(&corners)[dim], const bool colorize=false)
template<int dim>
void	parallelepiped (Triangulation< dim > &tria, const Point< dim >(&corners)[dim], const bool colorize=false)
template<int dim>
void	subdivided_parallelepiped (Triangulation< dim > &tria, const unsigned int n_subdivisions, const Point< dim >(&corners)[dim], const bool colorize=false)
template<int dim>
void	subdivided_parallelepiped (Triangulation< dim > &tria, const unsigned int(&n_subdivisions)[dim], const Point< dim >(&corners)[dim], const bool colorize=false)
template<int dim, int spacedim>
void	subdivided_parallelepiped (Triangulation< dim, spacedim > &tria, const Point< spacedim > &origin, const std::array< Tensor< 1, spacedim >, dim > &edges, const std::vector< unsigned int > &subdivisions={}, const bool colorize=false)
template<int dim>
void	enclosed_hyper_cube (Triangulation< dim > &tria, const double left=0., const double right=1., const double thickness=1., const bool colorize=false)
template<int dim>
void	hyper_ball (Triangulation< dim > &tria, const Point< dim > &center=Point< dim >(), const double radius=1., const bool attach_spherical_manifold_on_boundary_cells=false)
template<int dim>
void	hyper_ball_balanced (Triangulation< dim > &tria, const Point< dim > &center=Point< dim >(), const double radius=1.)
template<int spacedim>
void	hyper_sphere (Triangulation< spacedim - 1, spacedim > &tria, const Point< spacedim > &center=Point< spacedim >(), const double radius=1.)
template<int dim>
void	quarter_hyper_ball (Triangulation< dim > &tria, const Point< dim > &center=Point< dim >(), const double radius=1.)
template<int dim>
void	half_hyper_ball (Triangulation< dim > &tria, const Point< dim > &center=Point< dim >(), const double radius=1.)
template<int dim>
void	cylinder (Triangulation< dim > &tria, const double radius=1., const double half_length=1.)
template<int dim>
void	truncated_cone (Triangulation< dim > &tria, const double radius_0=1.0, const double radius_1=0.5, const double half_length=1.0)
template<int dim, int spacedim>
void	hyper_cross (Triangulation< dim, spacedim > &tria, const std::vector< unsigned int > &sizes, const bool colorize_cells=false)
	A center cell with stacks of cell protruding from each surface. More...
template<int dim>
void	hyper_L (Triangulation< dim > &tria, const double left=-1., const double right=1., const bool colorize=false)
template<int dim, int spacedim>
void	subdivided_hyper_L (Triangulation< dim, spacedim > &tria, const std::vector< unsigned int > &repetitions, const Point< dim > &bottom_left, const Point< dim > &top_right, const std::vector< int > &n_cells_to_remove)
template<int dim>
void	hyper_cube_slit (Triangulation< dim > &tria, const double left=0., const double right=1., const bool colorize=false)
template<int dim>
void	hyper_shell (Triangulation< dim > &tria, const Point< dim > &center, const double inner_radius, const double outer_radius, const unsigned int n_cells=0, bool colorize=false)
template<int dim>
void	eccentric_hyper_shell (Triangulation< dim > &triangulation, const Point< dim > &inner_center, const Point< dim > &outer_center, const double inner_radius, const double outer_radius, const unsigned int n_cells)
template<int dim>
void	half_hyper_shell (Triangulation< dim > &tria, const Point< dim > &center, const double inner_radius, const double outer_radius, const unsigned int n_cells=0, const bool colorize=false)
template<int dim>
void	quarter_hyper_shell (Triangulation< dim > &tria, const Point< dim > &center, const double inner_radius, const double outer_radius, const unsigned int n_cells=0, const bool colorize=false)
template<int dim>
void	cylinder_shell (Triangulation< dim > &tria, const double length, const double inner_radius, const double outer_radius, const unsigned int n_radial_cells=0, const unsigned int n_axial_cells=0)
template<int dim, int spacedim>
void	torus (Triangulation< dim, spacedim > &tria, const double R, const double r, const unsigned int n_cells_toroidal=6, const double phi=2.0 *numbers::PI)
template<int dim>
void	hyper_cube_with_cylindrical_hole (Triangulation< dim > &triangulation, const double inner_radius=.25, const double outer_radius=.5, const double L=.5, const unsigned int repetitions=1, const bool colorize=false)
template<int dim>
void	concentric_hyper_shells (Triangulation< dim > &triangulation, const Point< dim > &center, const double inner_radius=0.125, const double outer_radius=0.25, const unsigned int n_shells=1, const double skewness=0.1, const unsigned int n_cells_per_shell=0, const bool colorize=false)
void	moebius (Triangulation< 3, 3 > &tria, const unsigned int n_cells, const unsigned int n_rotations, const double R, const double r)
template<int dim, int spacedim>
void	generate_from_name_and_arguments (Triangulation< dim, spacedim > &tria, const std::string &grid_generator_function_name, const std::string &grid_generator_function_arguments)

Detailed Description

This namespace provides a collection of functions for generating triangulations for some basic geometries.

Some of these functions receive a flag colorize (see the glossary entry on colorization). If this is set, parts of the boundary receive different boundary indicators allowing them to be distinguished for the purpose of evaluating different boundary conditions.

If the domain is curved, each of the domain parts that should be refined by following an appropriate Manifold description will receive a different manifold indicator, and the correct Manifold descriptor will be attached to the Triangulation. Notice that if you later transform the triangulation, you have to make sure you attach the correct new Manifold to the triangulation.

Function Documentation

hyper_cube()

template<int dim, int spacedim>

void GridGenerator::hyper_cube	(	Triangulation< dim, spacedim > &	tria,
		const double	left = 0.,
		const double	right = 1.,
		const bool	colorize = false
	)

Initialize the given triangulation with a hypercube (line in 1D, square in 2D, etc) consisting of exactly one cell. The hypercube volume is the tensor product interval \([left,right]^{\text{dim}}\) in the present number of dimensions, where the limits are given as arguments. They default to zero and unity, then producing the unit hypercube.

If the argument colorize is false, then all boundary indicators are set to zero (the default boundary indicator) for 2d and 3d. If it is true, the boundary is colorized as in hyper_rectangle(). In 1d the indicators are always colorized, see hyper_rectangle().

If dim < spacedim, this will create a dim dimensional object in the first dim coordinate directions embedded into the spacedim dimensional space with the remaining entries set to zero. For example, a Triangulation<2,3> will be a square in the xy plane with z=0.

See also subdivided_hyper_cube() for a coarse mesh consisting of several cells. See hyper_rectangle(), if different lengths in different ordinate directions are required.

Precondition

The triangulation passed as argument needs to be empty when calling this function.

simplex()

template<int dim>

void GridGenerator::simplex	(	Triangulation< dim, dim > &	tria,
		const std::vector< Point< dim >> &	vertices
	)

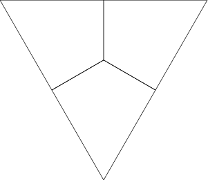
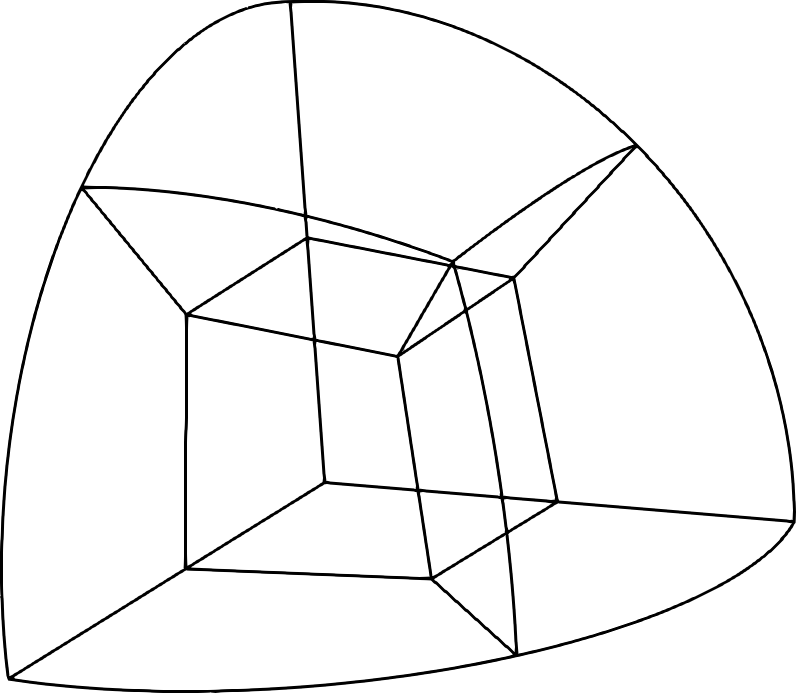
Create a \(d\)-simplex (i.e., a triangle in 2d, or a tetrahedron in 3d) with \(d+1\) corners. Since deal.II does not support triangular and tetrahedral cells, the simplex described by the input arguments is subdivided into quadrilaterals and hexahedra by adding edge, face, and simplex midpoints, resulting in a mesh that consists of \(d+1\) quadrilateral or hexahedral cells.

The vertices argument contains a vector with all d+1 vertices defining the corners of the simplex. They must be given in an order such that the vectors from the first vertex to each of the others form a right-handed system.

The meshes generated in two and three dimensions are:

Parameters

tria	The triangulation to be created. It needs to be empty upon calling this function.
vertices	The dim+1 corners of the simplex.

Note

Implemented for Triangulation<2,2>, Triangulation<3,3>.

Author

Guido Kanschat

subdivided_hyper_cube()

template<int dim, int spacedim>

void GridGenerator::subdivided_hyper_cube	(	Triangulation< dim, spacedim > &	tria,
		const unsigned int	repetitions,
		const double	left = 0.,
		const double	right = 1.,
		const bool	colorize = false
	)

Same as hyper_cube(), but with the difference that not only one cell is created but each coordinate direction is subdivided into repetitions cells. Thus, the number of cells filling the given volume is repetitionsdim.

If dim < spacedim, this will create a dim dimensional object in the first dim coordinate directions embedded into the spacedim dimensional space with the remaining entries set to zero. For example, a Triangulation<2,3> will be a square in the xy plane with z=0.

Precondition

The triangulation passed as argument needs to be empty when calling this function.

Parameters

tria	The triangulation to create. It needs to be empty upon calling this function.
repetitions	A vector of dim positive values denoting the number of cells to generate in that direction. For example, the first component of the vector specifies the number of cells in the x-direction, the second component specifies that for the y-direction for dim=2.
left	Lower bound for the interval used to create the hyper cube.
right	Upper bound for the interval used to create the hyper cube.
colorize	Assign different boundary ids if set to true.

hyper_rectangle()

template<int dim, int spacedim>

void GridGenerator::hyper_rectangle	(	Triangulation< dim, spacedim > &	tria,
		const Point< dim > &	p1,
		const Point< dim > &	p2,
		const bool	colorize = false
	)

Create a coordinate-parallel brick from the two diagonally opposite corner points p1 and p2.

If the colorize flag is true, then the boundary_ids of the boundary faces are assigned, such that the lower one in x-direction is 0, the upper one is 1. The indicators for the surfaces in y-direction are 2 and 3, the ones for z are 4 and 5. This corresponds to the numbers of faces of the unit square of cube as laid out in the documentation of the GeometryInfo class; see also the glossary entry on colorization. Importantly, however, in 3d colorization does not set boundary_ids of edges, but only of faces, because each boundary edge is shared between two faces and it is not clear how the boundary id of an edge should be set in that case.

Additionally, if colorize is true, material ids are assigned to the cells according to the octant their center is in: being in the right half space for any coordinate direction x_i adds 2ⁱ. For instance, a cell with center point (1,-1,1) yields a material id 5, assuming that the center of the hyper rectangle lies at the origin. No manifold id is set for the cells.

If dim < spacedim, this will create a dim dimensional object in the first dim coordinate directions embedded into the spacedim dimensional space with the remaining entries set to zero. For example, a Triangulation<2,3> will be a rectangle in the xy plane with z=0, defined by the two opposing corners p1 and p2.

Precondition

The triangulation passed as argument needs to be empty when calling this function.

subdivided_hyper_rectangle() [1/3]

template<int dim, int spacedim>

void GridGenerator::subdivided_hyper_rectangle	(	Triangulation< dim, spacedim > &	tria,
		const std::vector< unsigned int > &	repetitions,
		const Point< dim > &	p1,
		const Point< dim > &	p2,
		const bool	colorize = false
	)

Create a coordinate-parallel brick from the two diagonally opposite corner points p1 and p2. The number of cells in coordinate direction i is given by the integer repetitions[i].

To get cells with an aspect ratio different from that of the domain, use different numbers of subdivisions, given by repetitions, in different coordinate directions. The minimum number of subdivisions in each direction is 1.

If the colorize flag is true, then the boundary_ids of the surfaces are assigned, such that the lower one in x-direction is 0, the upper one is 1 (the left and the right vertical face). The indicators for the surfaces in y-direction are 2 and 3, the ones for z are 4 and 5. Additionally, material ids are assigned to the cells according to the octant their center is in: being in the right half plane for any coordinate direction x_i adds 2ⁱ (see the glossary entry on colorization). For instance, the center point (1,-1,1) yields a material id 5 (this means that in 2d only material ids 0,1,2,3 are assigned independent from the number of repetitions).

Note that the colorize flag is ignored in 1d and is assumed to always be true. That means the boundary indicator is 0 on the left and 1 on the right. See step-15 for details.

If dim < spacedim, this will create a dim dimensional object in the first dim coordinate directions embedded into the spacedim dimensional space with the remaining entries set to zero. For example, a Triangulation<2,3> will be a rectangle in the xy plane with z=0, defined by the two opposing corners p1 and p2.

Note

For an example of the use of this function see the step-28 tutorial program.

Parameters

tria	The triangulation to be created. It needs to be empty upon calling this function.
repetitions	A vector of dim positive values denoting the number of cells to generate in that direction.
p1	First corner point.
p2	Second corner opposite to p1.
colorize	Assign different boundary ids if set to true. The same comments apply as for the hyper_rectangle() function.

subdivided_hyper_rectangle() [2/3]

template<int dim>

void GridGenerator::subdivided_hyper_rectangle	(	Triangulation< dim > &	tria,
		const std::vector< std::vector< double >> &	step_sizes,
		const Point< dim > &	p_1,
		const Point< dim > &	p_2,
		const bool	colorize = false
	)

Like the previous function. However, here the second argument does not denote the number of subdivisions in each coordinate direction, but a sequence of step sizes for each coordinate direction. The domain will therefore be subdivided into step_sizes[i].size() cells in coordinate direction i, with width step_sizes[i][j] for the jth cell.

This function is therefore the right one to generate graded meshes where cells are concentrated in certain areas, rather than a uniformly subdivided mesh as the previous function generates.

The step sizes have to add up to the dimensions of the hyper rectangle specified by the points p1 and p2.

subdivided_hyper_rectangle() [3/3]

template<int dim>

void GridGenerator::subdivided_hyper_rectangle	(	Triangulation< dim > &	tria,
		const std::vector< std::vector< double >> &	spacing,
		const Point< dim > &	p,
		const Table< dim, types::material_id > &	material_id,
		const bool	colorize = false
	)

Like the previous function, but with the following twist: the material_id argument is a dim-dimensional array that, for each cell, indicates which material_id should be set. In addition, and this is the major new functionality, if the material_id of a cell is (unsigned char)(-1), then that cell is deleted from the triangulation, i.e. the domain will have a void there.

Note

If you need a lot of holes, you may consider cheese().

cheese()

template<int dim, int spacedim>

void GridGenerator::cheese	(	Triangulation< dim, spacedim > &	tria,
		const std::vector< unsigned int > &	holes
	)

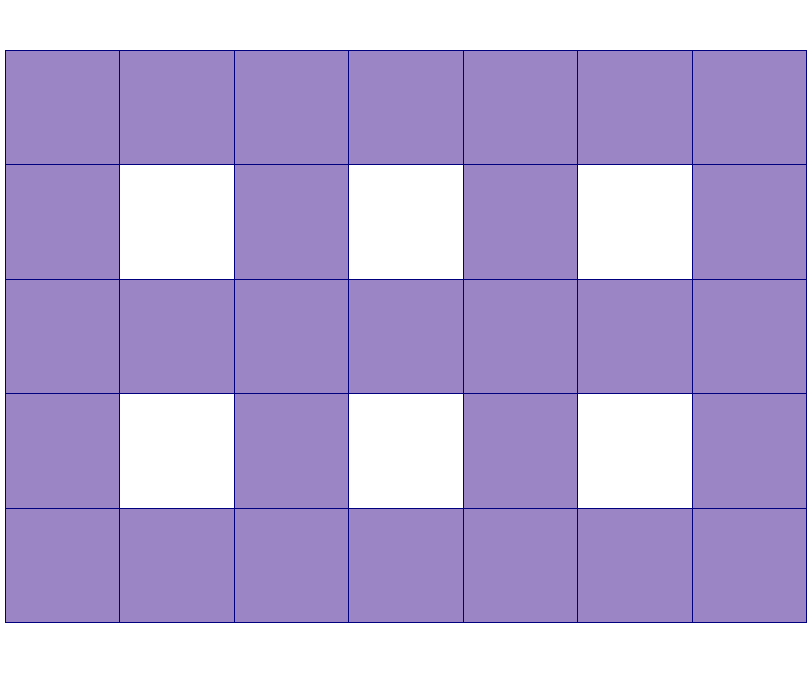
Rectangular domain with rectangular pattern of holes.

The domain itself is rectangular, very much as if it had been generated by subdivided_hyper_rectangle(). The argument holes specifies how many square holes the domain should have in each coordinate direction. The total number of mesh cells in that direction is then twice this number plus one.

The number of holes in one direction must be at least one.

An example with two by three holes is

If dim < spacedim, this will create a dim dimensional object in the first dim coordinate directions embedded into the spacedim dimensional space with the remaining entries set to zero.

Parameters

tria	The triangulation to be created. It needs to be empty upon calling this function.
holes	Positive number of holes in each of the dim directions.

Author

Guido Kanschat

plate_with_a_hole()

template<int dim>

void GridGenerator::plate_with_a_hole	(	Triangulation< dim > &	tria,
		const double	inner_radius = 0.4,
		const double	outer_radius = 1.,
		const double	pad_bottom = 2.,
		const double	pad_top = 2.,
		const double	pad_left = 1.,
		const double	pad_right = 1.,
		const Point< dim >	center = Point< dim >(),
		const types::manifold_id	polar_manifold_id = 0,
		const types::manifold_id	tfi_manifold_id = 1,
		const double	L = 1.,
		const unsigned int	n_slices = 2,
		const bool	colorize = false
	)

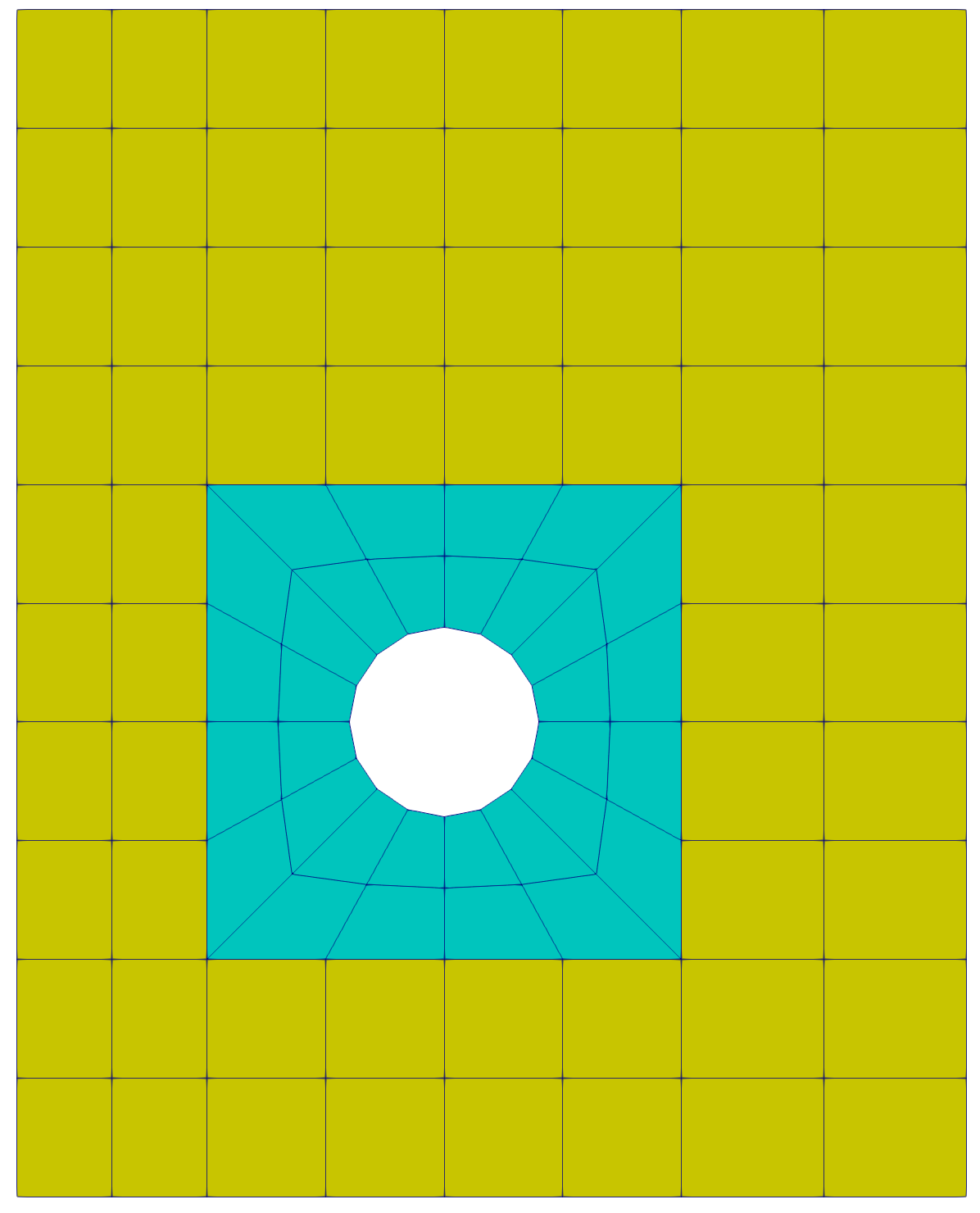
Rectangular plate with an (offset) cylindrical hole.

Generate a rectangular plate with an (offset) cylindrical hole. The geometry consists of 2 regions: The first is a square region with length outer_radius and a hole of radius inner_radius . Cells in this region will have TransfiniteInterpolationManifold with manifold id tfi_manifold_id attached to them. Additionally, the boundary faces of the hole will be associated with a PolarManifold (in 2D) or CylindricalManifold (in 3D). The center of this region can be prescribed via center , namely the axis of the hole will be located at center . The second region describes the remainder of the bulk material. It is specified via padding parameters pad_bottom, padding_top, padding_left and padding_right. All cells in this region will have a FlatManifold attached to them. The final width of the plate will be padding_left + 2*outer_radius + padding_right, while its length is padding_top + 2*outer_radius + padding_bottom.

Here is the non-symmetric grid (after one global refinement, colored according to manifold id) in 2D and 3D, respectively:

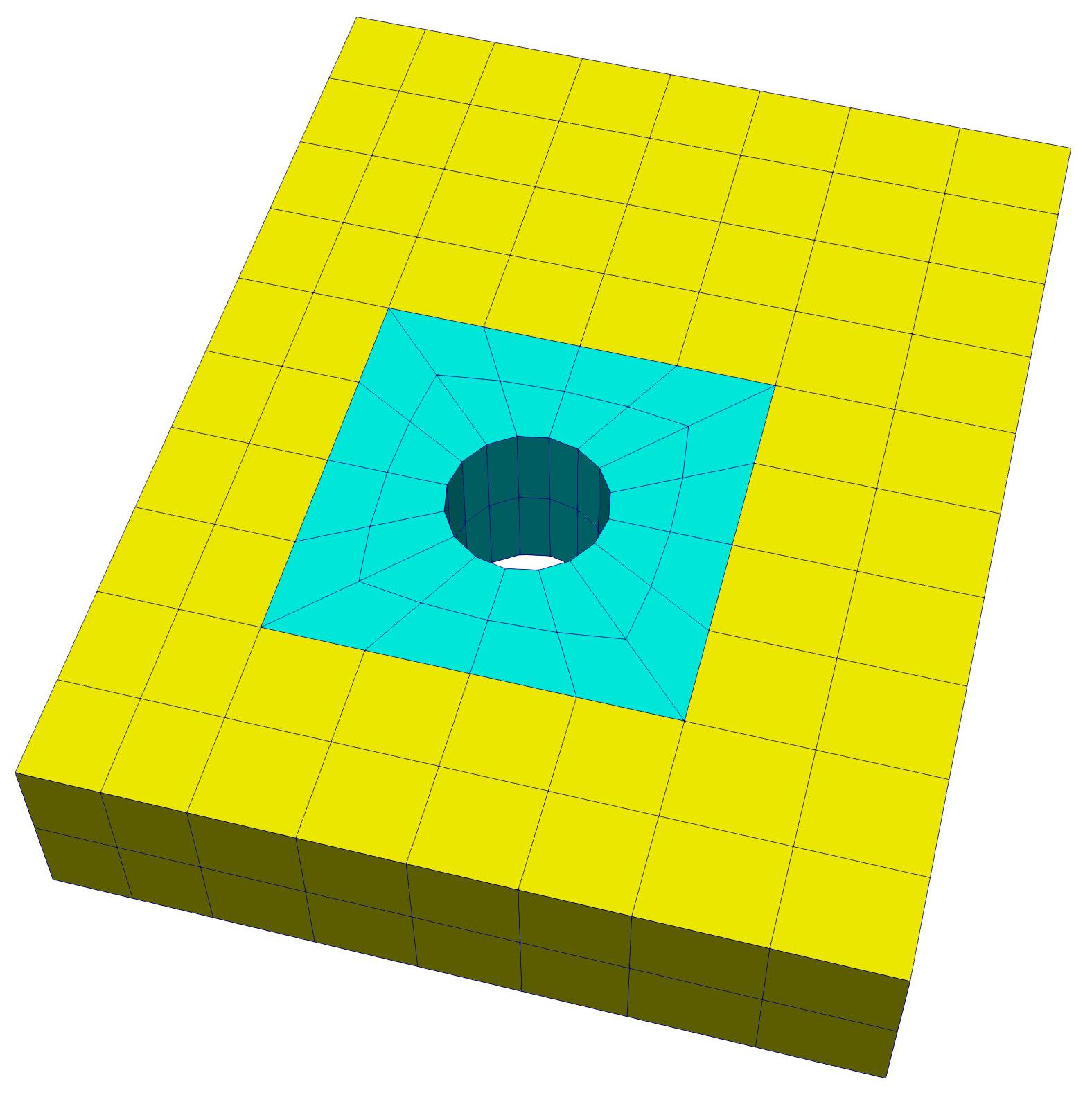
In 3D, triangulation will be extruded in the z-direction by the total height of L using n_slices slices (minimum is 2).

If the colorize flag is true, the boundary_ids of the boundary faces are assigned such that the lower one in the x-direction is 0, and the upper one is 1 (see the glossary entry on colorization). The indicators for the surfaces in the y-direction are 2 and 3, and the ones for the z-direction are 5 and 6. The hole boundary has indicator 4.

tria is the triangulation to be created. It needs to be empty upon calling this function.

Author

Denis Davydov, 2018

channel_with_cylinder()

template<int dim>

void GridGenerator::channel_with_cylinder	(	Triangulation< dim > &	tria,
		const double	shell_region_width = 0.03,
		const unsigned int	n_shells = 2,
		const double	skewness = 2.0,
		const bool	colorize = false
	)

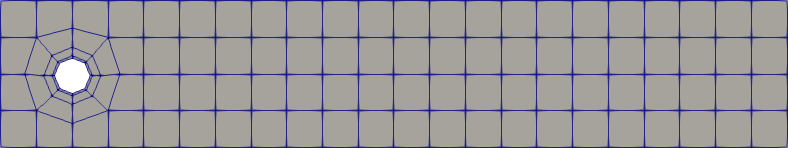
Generate a grid consisting of a channel with a cylinder. This is a common benchmark for Navier-Stokes solvers. The geometry consists of a channel of size \([0, 2.2] \times [0, 0.41] \times [0, 0.41] \) (where the \(z\) dimension is omitted in 2D) with a cylinder, parallel to the \(z\) axis with diameter \(0.1\), centered at \((0.2, 0.2, 0)\). The channel has three distinct regions:

1. If n_shells is greater than zero, then there are that many shells centered around the cylinder,
2. a blending region between the shells and the rest of the triangulation, and
3. a bulk region consisting of Cartesian cells.

Since the cylinder is slightly offset from the center of the channel, this geometry results in vortex shedding at moderate Reynolds numbers. Here is the grid (without additional global refinement) in 2D:

and in 3D:

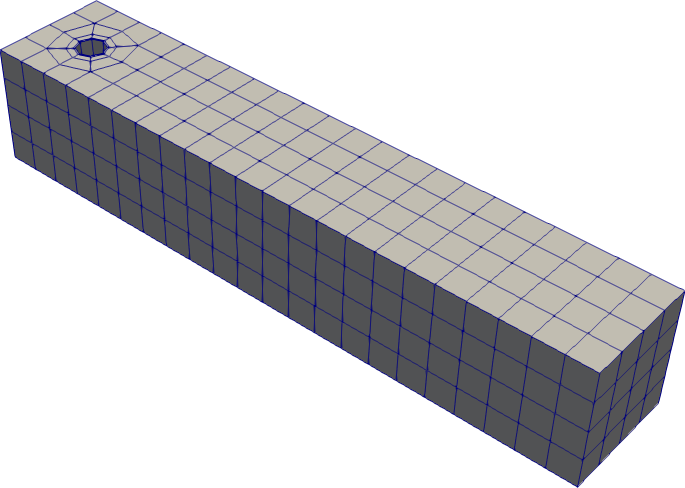
The resulting Triangulation uses three manifolds: a PolarManifold (in 2D) or CylindricalManifold (in 3D) with manifold id \(0\), a TransfiniteInterpolationManifold with manifold id \(1\), and a FlatManifold everywhere else. For more information on this topic see the glossary entry on manifold indicators. The cell faces on the cylinder and surrounding shells have manifold ids of \(0\), while the cell volumes adjacent to the shells (or, if they do not exist, the cylinder) have a manifold id of \(1\). Put another way: this grid uses TransfiniteInterpolationManifold to smoothly transition from the shells (generated with GridGenerator::concentric_hyper_shells) to the bulk region. All other cell volumes and faces have manifold id numbers::flat_manifold_id and use FlatManifold. All cells with id numbers::flat_manifold_id are rectangular prisms aligned with the coordinate axes.

The picture below shows part of the 2D grid (using all default arguments to this function) after two global refinements. The cells with manifold id \(0\) are orange (the polar manifold id), cells with manifold id \(1\) are yellow (the transfinite interpolation manifold id), and the ones with manifold id numbers::flat_manifold_id are cyan:

Parameters

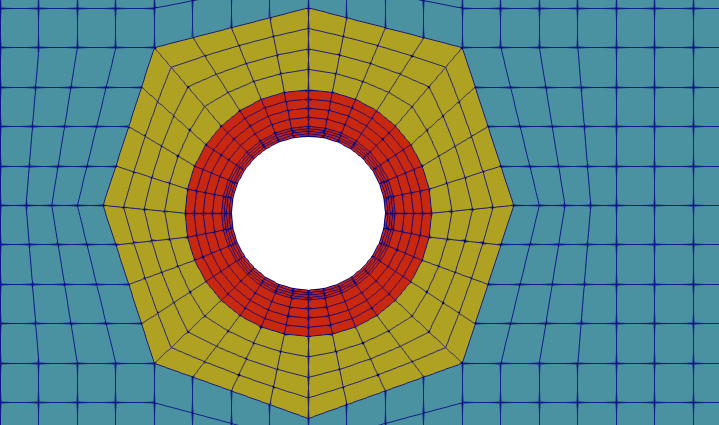
tria	Triangulation to be created. Must be empty upon calling this function.
shell_region_width	Width of the layer of shells around the cylinder. This value should be between \(0\) and \(0.05\); the default value is \(0.03\).
n_shells	Number of shells to use in the shell layer.
skewness	Parameter controlling how close the shells are to the cylinder: see the mathematical definition given in GridGenerator::concentric_hyper_shells.
colorize	Assign different boundary ids if set to true. For more information on boundary indicators see this glossary entry. The left boundary (at \(x = 0\)) is assigned an id of \(0\), the right boundary (at \(x = 2.2\)) is assigned an id of \(1\), the cylinder boundary is assigned an id of \(2\), and the channel walls are assigned an id of \(3\).

See the original paper for more information:

@inbook{schafer1996,
author    = {Sch{\"a}fer, M. and Turek, S. and Durst, F. and Krause, E.
             and Rannacher, R.},
title     = {Benchmark Computations of Laminar Flow Around a Cylinder},
bookTitle = {Flow Simulation with High-Performance Computers II: DFG
             Priority Research Programme Results 1993--1995},
year      = {1996},
publisher = {Vieweg+Teubner Verlag},
address   = {Wiesbaden},
pages     = {547--566},
isbn      = {978-3-322-89849-4},
doi       = {10.1007/978-3-322-89849-4_39},
url       = {https://doi.org/10.1007/978-3-322-89849-4_39}
}

general_cell()

template<int dim, int spacedim>

void GridGenerator::general_cell	(	Triangulation< dim, spacedim > &	tria,
		const std::vector< Point< spacedim >> &	vertices,
		const bool	colorize = false
	)

A general dim -dimensional cell (a segment if dim is 1, a quadrilateral if dim is 2, or a hexahedron if dim is 3) immersed in a spacedim -dimensional space. It is the responsibility of the user to provide the vertices in the right order (see the documentation of the GeometryInfo class) because the vertices are stored in the same order as they are given. It is also important to make sure that the volume of the cell is positive.

If the argument colorize is false, then all boundary indicators are set to zero for 2d and 3d. If it is true, the boundary is colorized as in hyper_rectangle() (see the glossary entry on colorization). In 1d the indicators are always colorized, see hyper_rectangle().

Parameters

tria	The triangulation that will be created
vertices	The 2^dim vertices of the cell
colorize	If true, set different boundary ids.

Author

Bruno Turcksin

parallelogram()

template<int dim>

void GridGenerator::parallelogram	(	Triangulation< dim > &	tria,
		const Point< dim >(&)	corners[dim],
		const bool	colorize = false
	)

A parallelogram. The first corner point is the origin. The next dim vertices are the ones given in the second argument and the last vertex will be the sum of the two vectors connecting the origin to those points. Colorizing is done in the same way as in hyper_rectangle().

Note

This function is implemented in 2d only.

Parameters

tria	The triangulation to be created. It needs to be empty upon calling this function.
corners	Second and third vertices of the parallelogram.
colorize	Assign different boundary ids if true. (see the glossary entry on colorization).

parallelepiped()

template<int dim>

void GridGenerator::parallelepiped	(	Triangulation< dim > &	tria,
		const Point< dim >(&)	corners[dim],
		const bool	colorize = false
	)

A parallelepiped. The first corner point is the origin. The dim adjacent points are vectors describing the edges of the parallelepiped with respect to the origin. Additional points are sums of these dim vectors. Colorizing is done according to hyper_rectangle().

Note

This function silently reorders the vertices on the cells to lexicographic ordering (see GridReordering::reorder_grid). In other words, if reordering of the vertices does occur, the ordering of vertices in the array of corners will no longer refer to the same triangulation.

Precondition

The triangulation passed as argument needs to be empty when calling this function.

subdivided_parallelepiped() [1/3]

template<int dim>

void GridGenerator::subdivided_parallelepiped	(	Triangulation< dim > &	tria,
		const unsigned int	n_subdivisions,
		const Point< dim >(&)	corners[dim],
		const bool	colorize = false
	)

A subdivided parallelepiped. The first corner point is the origin. The dim adjacent points are vectors describing the edges of the parallelepiped with respect to the origin. Additional points are sums of these dim vectors. The variable n_subdivisions designates the number of subdivisions in each of the dim directions. Colorizing is done according to hyper_rectangle().

Precondition

The triangulation passed as argument needs to be empty when calling this function.

subdivided_parallelepiped() [2/3]

template<int dim>

void GridGenerator::subdivided_parallelepiped	(	Triangulation< dim > &	tria,
		const unsigned int(&)	n_subdivisions[dim],
		const Point< dim >(&)	corners[dim],
		const bool	colorize = false
	)

A subdivided parallelepiped, i.e., the same as above, but where the number of subdivisions in each of the dim directions may vary. Colorizing is done according to hyper_rectangle().

Precondition

The triangulation passed as argument needs to be empty when calling this function.

subdivided_parallelepiped() [3/3]

template<int dim, int spacedim>

void GridGenerator::subdivided_parallelepiped	(	Triangulation< dim, spacedim > &	tria,
		const Point< spacedim > &	origin,
		const std::array< Tensor< 1, spacedim >, dim > &	edges,
		const std::vector< unsigned int > &	subdivisions = {},
		const bool	colorize = false
	)

A subdivided parallelepiped.

Parameters

tria	The triangulation to be created. It needs to be empty upon calling this function.
origin	First corner of the parallelepiped.
edges	An array of dim tensors describing the length and direction of the edges from origin.
subdivisions	Number of subdivisions in each of the dim directions. Each entry must be positive. An empty vector is equivalent to one subdivision in each direction.
colorize	Assign different boundary ids if set to true (see the glossary entry on colorization).

Note

Implemented for all combinations of dim and spacedim.

You likely need to help the compiler by explicitly specifying the two template parameters when calling this function.

enclosed_hyper_cube()

template<int dim>

void GridGenerator::enclosed_hyper_cube	(	Triangulation< dim > &	tria,
		const double	left = 0.,
		const double	right = 1.,
		const double	thickness = 1.,
		const bool	colorize = false
	)

Hypercube with a layer of hypercubes around it. Parameters left and right give the lower and upper bound of the inner hypercube in all coordinate directions. thickness marks the size of the layer cells.

If the flag colorize is set, the outer cells get material ids according to the following scheme: extending over the inner cube in (+/-) x-direction 1/2, y-direction 4/8, z-direction 16/32. A bitwise OR operation is used to get these values at the corners and edges (3d), (see also the glossary entry on colorization).

Presently only available in 2d and 3d.

Precondition

The triangulation passed as argument needs to be empty when calling this function.

hyper_ball()

template<int dim>

void GridGenerator::hyper_ball	(	Triangulation< dim > &	tria,
		const Point< dim > &	center = Point< dim >(),
		const double	radius = 1.,
		const bool	attach_spherical_manifold_on_boundary_cells = false
	)

Initialize the given triangulation with several coarse mesh cells that cover a hyperball, i.e. a circle in 2d or a ball in 3d, around center with given radius. The function is used in step-6.

In order to avoid degenerate cells at the boundaries, the circle is triangulated by five cells, whereas in 3d the ball is subdivided by seven cells. Specifically, these cells are one cell in the center plus one "cap" cell on each of the faces of this center cell. This ensures that under repeated refinement, none of the cells at the outer boundary will degenerate to have an interior angle approaching 180 degrees, as opposed to the case where one might start with just one square (or cube) to approximate the domain. The diameter of the center cell is chosen so that the aspect ratio of the boundary cells after one refinement is optimized.

This function is declared to exist for triangulations of all space dimensions, but throws an error if called in 1d.

By default, the manifold_id is set to 0 on the boundary faces, 1 on the boundary cells, and types::flat_manifold_id on the central cell and on internal faces.

A SphericalManifold is attached by default to the boundary faces for correct placement of boundary vertices upon refinement and to be able to use higher order mappings. However, it turns out that this strategy may not be the optimal one to create a good a mesh for a hyperball. The "Possibilities for extensions" section of step-6 has an extensive discussion of how one would construct better meshes and what one needs to do for it. Setting the argument attach_spherical_manifold_on_boundary_cells to true attaches a SphericalManifold manifold also to the cells adjacent to the boundary, and not only to the boundary faces.

Note

Since this is likely one of the earliest functions users typically consider to create meshes with curved boundaries, let us also comment on one aspect that is often confusing: Namely, that what one sees is not always what is actually happening. Specifically, if you output the coarse mesh with a function such as GridOut::write_vtk() using default options, then one doesn't generally get to see curved faces at the boundary. That's because most file formats by default only store vertex locations, with the implicit understanding that cells are composed from these vertices and bounded by straight edges. At the same time, the fact that this function attaches a SphericalManifold object to the boundary faces means that at least internally, edges really are curved. If you want to see them that way, you need to make sure that the function you use to output the mesh actually plots boundary faces as curved lines rather than straight lines characterized by only the locations of the two end points. For example, GridOut::write_gnuplot() can do that if you set the corresponding flag in the GridOutFlags::Gnuplot structure. It is, however, an entirely separate consideration whether you are actually computing on curved cells. In typical finite element computations, one has to compute integrals and these are computed by transforming back actual cells using a mapping to the reference cell. What mapping is used determines what shape the cells have for these internal computations: For example, with the widely used \(Q_1\) mapping (implicitly used in step-6), integration always happens on cells that are assumed to have straight boundaries described by only the vertex locations. In other words, if such a mapping is used, then the cells of the domain really do have straight edges, regardless of the manifold description attached to these edges and regardless of the flags given when generating output. As a consequence of all of this, it is important to distinguish three things: (i) the manifold description attached to an object in the mesh; (ii) the mapping used in integration; and (iii) the style used in outputting graphical information about the mesh. All of these can be chosen more or less independently of each other, and what you see visualized is not necessarily exactly what is happening.

Precondition

The triangulation passed as argument needs to be empty when calling this function.

hyper_ball_balanced()

template<int dim>

void GridGenerator::hyper_ball_balanced	(	Triangulation< dim > &	tria,
		const Point< dim > &	center = Point< dim >(),
		const double	radius = 1.
	)

This is an alternative to hyper_ball with 12 cells in 2d and 32 cells in 3d, which provides a better balance between the size of the cells around the outer curved boundaries and the cell in the interior. The mesh is based on the cells used by GridGenerator::quarter_hyper_ball() with appropriate copies and rotations to fill the whole ball.

The following pictures show the resulting mesh in 2D (left) and 3D:

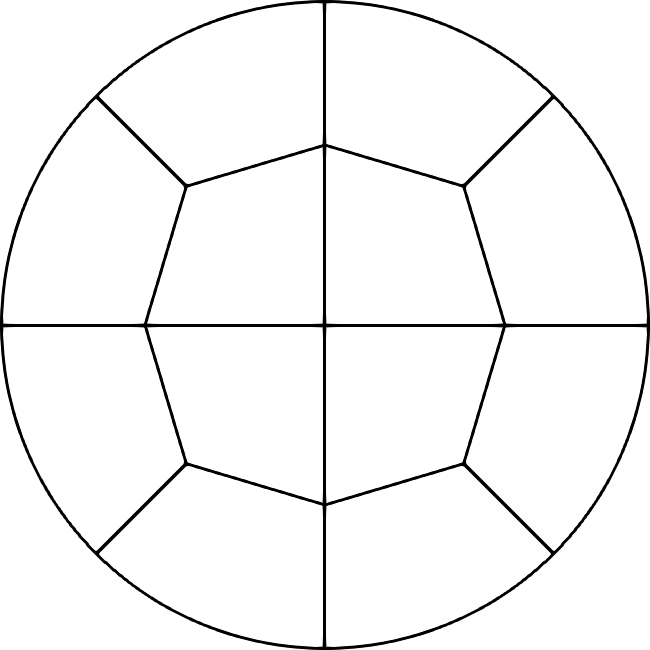
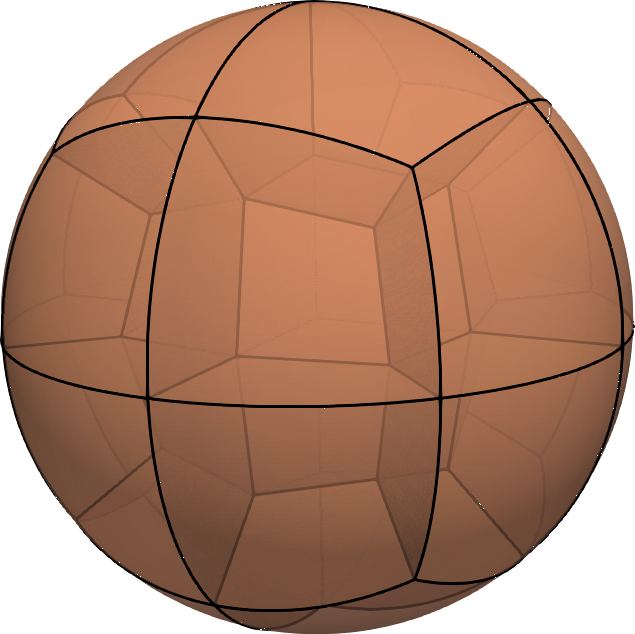
By default, the manifold_id is set to 0 on the boundary faces, 1 on the boundary cells, and types::flat_manifold_id on the central cell and on internal faces.

Precondition

The triangulation passed as argument needs to be empty when calling this function.

hyper_sphere()

template<int spacedim>

void GridGenerator::hyper_sphere	(	Triangulation< spacedim - 1, spacedim > &	tria,
		const Point< spacedim > &	center = Point< spacedim >(),
		const double	radius = 1.
	)

Creates a hyper sphere, i.e., a surface of a ball in spacedim dimensions. This function only exists for dim+1=spacedim in 2 and 3 space dimensions. (To create a mesh of a ball, use GridGenerator::hyper_ball().)

By default, all manifold ids of the triangulation are set to zero, and a SphericalManifold is attached to the grid.

The following pictures are generated with:

Triangulation<2,3>   triangulation;
GridGenerator::hyper_sphere(triangulation);
triangulation.refine_global(3);

See the documentation module on manifolds for more details.

Precondition

The triangulation passed as argument needs to be empty when calling this function.

quarter_hyper_ball()

template<int dim>

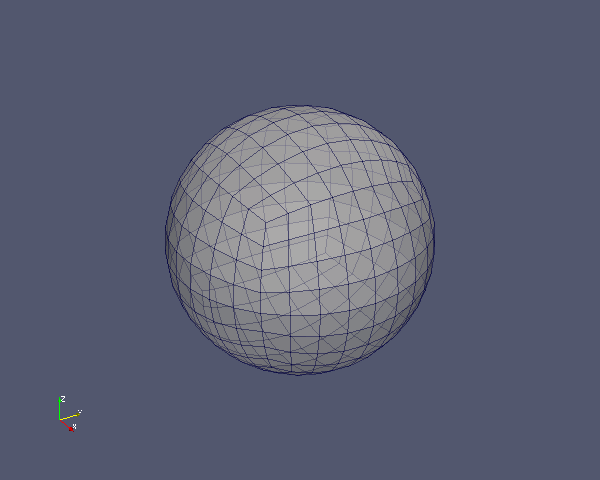
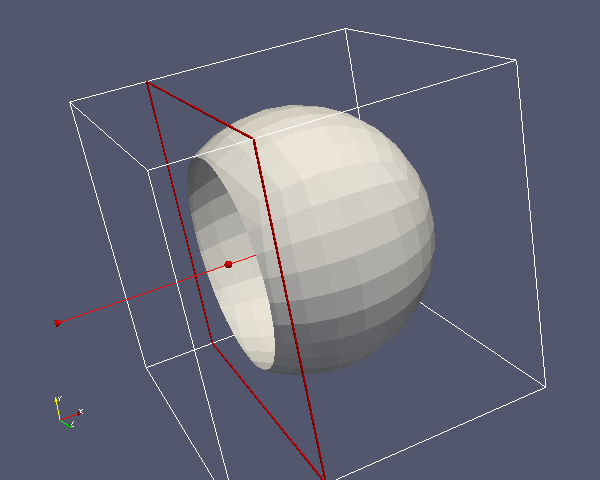
void GridGenerator::quarter_hyper_ball	(	Triangulation< dim > &	tria,
		const Point< dim > &	center = Point< dim >(),
		const double	radius = 1.
	)

This class produces a hyper-ball intersected with the positive orthant relative to center, which contains three elements in 2d and four in 3d. The interior points of the mesh are chosen to balance the minimal singular value of the Jacobian of the mapping from reference to real coordinates among the cells around the interior point, which corresponds to a high mesh quality.

The boundary indicators for the final triangulation are 0 for the curved boundary and 1 for the cut plane. The manifold id for the curved boundary is set to zero, and a SphericalManifold is attached to it.

The resulting grid in 2D and 3D looks as follows:

Precondition

The triangulation passed as argument needs to be empty when calling this function.

half_hyper_ball()

template<int dim>

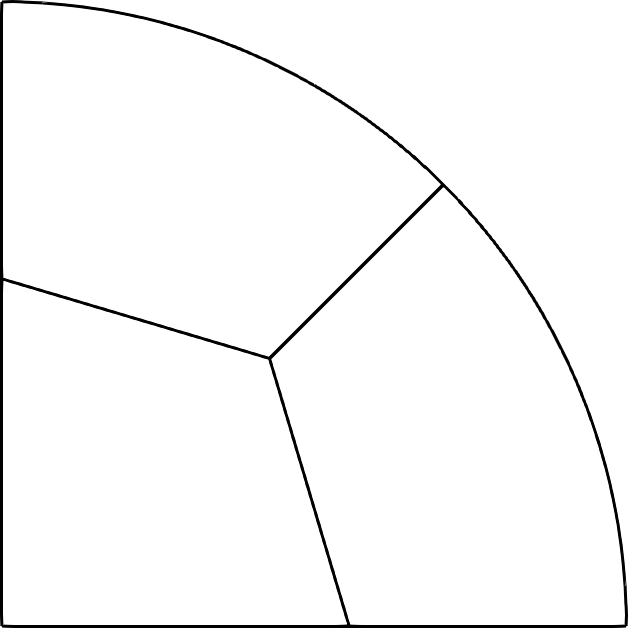
void GridGenerator::half_hyper_ball	(	Triangulation< dim > &	tria,
		const Point< dim > &	center = Point< dim >(),
		const double	radius = 1.
	)

This class produces a half hyper-ball around center, which contains four elements in 2d and 6 in 3d. The cut plane is perpendicular to the x-axis.

The boundary indicators for the final triangulation are 0 for the curved boundary and 1 for the cut plane. The manifold id for the curved boundary is set to zero, and a SphericalManifold is attached to it.

Precondition

The triangulation passed as argument needs to be empty when calling this function.

cylinder()

template<int dim>

void GridGenerator::cylinder	(	Triangulation< dim > &	tria,
		const double	radius = 1.,
		const double	half_length = 1.
	)

Create a dim dimensional cylinder where the \(x\)-axis serves as the axis of the cylinder. For the purposes of this function, a cylinder is defined as a (dim - 1) dimensional disk of given radius, extruded along the axis of the cylinder (which is the first coordinate direction). Consequently, in three dimensions, the cylinder extends from x=-half_length to x=+half_length and its projection into the yz-plane is a circle of radius radius. In two dimensions, the cylinder is a rectangle from x=-half_length to x=+half_length and from y=-radius to y=radius.

The boundaries are colored according to the following scheme: 0 for the hull of the cylinder, 1 for the left hand face and 2 for the right hand face (see the glossary entry on colorization).

The manifold id for the hull of the cylinder is set to zero, and a CylindricalManifold is attached to it.

Precondition

The triangulation passed as argument needs to be empty when calling this function.

truncated_cone()

template<int dim>

void GridGenerator::truncated_cone	(	Triangulation< dim > &	tria,
		const double	radius_0 = 1.0,
		const double	radius_1 = 0.5,
		const double	half_length = 1.0
	)

Create a cut cone around the x-axis. The cone extends from x=-half_length to x=half_length and its projection into the yz-plane is a circle of radius radius_0 at x=-half_length and a circle of radius radius_1 at x=+half_length. In between the radius is linearly decreasing.

In two dimensions, the cone is a trapezoid from x=-half_length to x=+half_length and from y=-radius_0 to y=radius_0 at x=-half_length and from y=-radius_1 to y=radius_1 at x=+half_length. In between the range of y is linearly decreasing.

The boundaries are colored according to the following scheme: 0 for the hull of the cone, 1 for the left hand face, and 2 for the right hand face (see the glossary entry on colorization). Both the boundary indicators and the manifold indicators are set.

In three dimensions, the manifold id of the hull is set to zero, and a CylindricalManifold is attached to it.

Here are the grids in 2D and 3D after two mesh refinements:

Precondition

The triangulation passed as argument needs to be empty when calling this function.

Author

Markus Bürg, 2009

hyper_cross()

template<int dim, int spacedim>

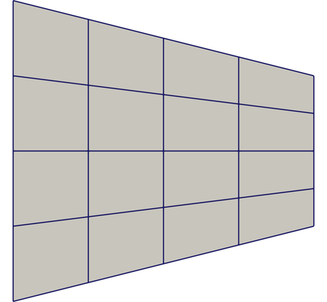
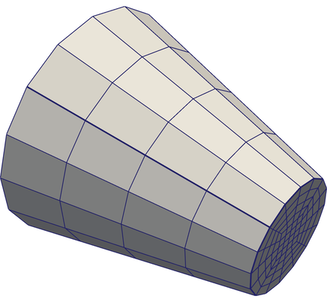
void GridGenerator::hyper_cross	(	Triangulation< dim, spacedim > &	tria,
		const std::vector< unsigned int > &	sizes,
		const bool	colorize_cells = false
	)

A center cell with stacks of cell protruding from each surface.

Each of the square mesh cells is Cartesian and has size one in each coordinate direction. The center of cell number zero is the origin.

Parameters

tria	A Triangulation object which has to be empty.
sizes	A vector of integers of dimension GeometryInfo<dim>::faces_per_cell with the following meaning: the legs of the cross are stacked on the faces of the center cell, in the usual order of deal.II cells, namely first \(-x\), then \(x\), then \(-y\) and so on. The corresponding entries in sizes name the number of cells stacked on this face. All numbers may be zero, thus L- and T-shaped domains are specializations of this domain.
colorize_cells	If colorization is enabled, then the material id of a cells corresponds to the leg it is in. The id of the center cell is zero, and then the legs are numbered starting at one (see the glossary entry on colorization).

Examples in two and three dimensions are:

Author

Guido Kanschat

hyper_L()

template<int dim>

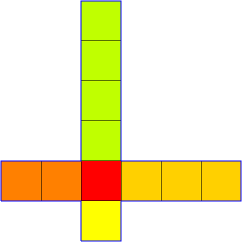
void GridGenerator::hyper_L	(	Triangulation< dim > &	tria,
		const double	left = -1.,
		const double	right = 1.,
		const bool	colorize = false
	)

Initialize the given triangulation with a hyper-L (in 2d or 3d) consisting of exactly 2^dim-1 cells. It produces the hypercube with the interval [left,right] without the hypercube made out of the interval [(left+right)/2,right] for each coordinate. Because the domain is about the simplest one with a reentrant (i.e., non-convex) corner, solutions of many partial differential equations have singularities at this corner. That is, at the corner, the gradient or a higher derivative (depending on the boundary conditions chosen) does not remain bounded. As a consequence, this domain is often used to test convergence of schemes when the solution lacks regularity.

If the colorize flag is true, the boundary_ids of the surfaces are assigned such that the left boundary is 0 and the others are assigned counterclockwise in ascending order (see the glossary entry on colorization). The colorize option only works in two dimensions.

This function will create the classical L-shape in 2d and it will look like the following in 3d:

Note

The 3d domain is also often referred to as the "Fichera corner", named after Gaetano Fichera (1922-1996) who first computed an approximation of the corner singularity exponent of the lowest eigenfunction of the domain.

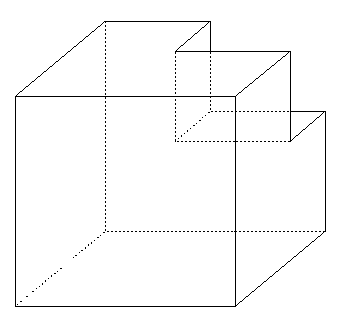
This function exists for triangulations of all space dimensions, but throws an error if called in 1d.

Precondition

The triangulation passed as argument needs to be empty when calling this function.

subdivided_hyper_L()

template<int dim, int spacedim>

void GridGenerator::subdivided_hyper_L	(	Triangulation< dim, spacedim > &	tria,
		const std::vector< unsigned int > &	repetitions,
		const Point< dim > &	bottom_left,
		const Point< dim > &	top_right,
		const std::vector< int > &	n_cells_to_remove
	)

Initialize the given triangulation in 2D or 3D with a generalized subdivided hyper-L.

This function produces a subdivided hyper rectangle with dimensions given by bottom_left and top_right, with the given number of subdivisions in each direction given in the vector repetitions, and with a number of cells removed, given in the vector n_cells_to_remove. Note that n_cells_to_remove contains integers, meaning that its entries can be both positive and negative. A positive number denotes cutting away cells in the 'positive' orientation, for example left to right in the x-direction, bottom to top in the y-direction, and front to back in the z-direction. A negative number denotes cutting away cells in the reverse direction, so right to left, top to bottom, and back to front.

This function may be used to generate a mesh for a backward facing step, a useful domain for benchmark problems in fluid dynamics. The first image is a backward facing step in 3D, generated by removing all cells in the z-direction, and 2 cells in the positive x- and y-directions:

And in 2D, we can cut away 1 cell in the negative x-direction, and 2 cells in the negative y-direction:

Note

This function is declared to exist for triangulations of all space dimensions, but throws an error if called in 1D.

Author

Mae Markowski, 2019

hyper_cube_slit()

template<int dim>

void GridGenerator::hyper_cube_slit	(	Triangulation< dim > &	tria,
		const double	left = 0.,
		const double	right = 1.,
		const bool	colorize = false
	)

Initialize the given Triangulation with a hypercube with a slit. In each coordinate direction, the hypercube extends from left to right.

In 2d, the split goes in vertical direction from x=(left+right)/2, y=left to the center of the square at x=y=(left+right)/2.

In 3d, the 2d domain is just extended in the z-direction, such that a plane cuts the lower half of a rectangle in two. This function is declared to exist for triangulations of all space dimensions, but throws an error if called in 1d.

If colorize is set to true, the faces forming the slit are marked with boundary id 1 and 2, respectively (see the glossary entry on colorization).

Precondition

The triangulation passed as argument needs to be empty when calling this function.

hyper_shell()

template<int dim>

void GridGenerator::hyper_shell	(	Triangulation< dim > &	tria,
		const Point< dim > &	center,
		const double	inner_radius,
		const double	outer_radius,
		const unsigned int	n_cells = 0,
		bool	colorize = false
	)

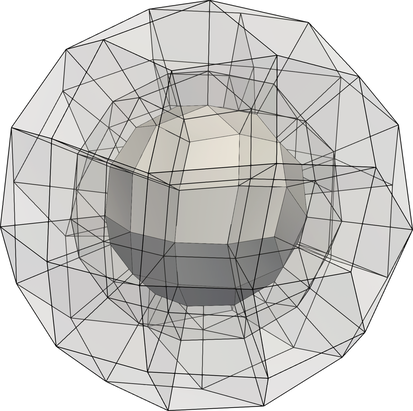
Produce a hyper-shell, the region between two spheres around center, with given inner_radius and outer_radius. The number n_cells indicates the number of cells of the resulting triangulation, i.e., how many cells form the ring (in 2d) or the shell (in 3d).

If the flag colorize is true, then the outer boundary will have the indicator 1 while the inner boundary has id zero. In 3d, this applies to both the faces and the edges of these boundaries. If the flag is false, both have indicator zero (see the glossary entry on colorization).

All manifold ids are set to zero, and a SphericalManifold is attached to every cell and face of the triangulation.

In 2d, the number n_cells of elements for this initial triangulation can be chosen arbitrarily. If the number of initial cells is zero (as is the default), then it is computed adaptively such that the resulting elements have the least aspect ratio.

In 3d, only certain numbers are allowed:

• 6 (or the default 0) for a surface based on a hexahedron (i.e. 6 panels on the inner sphere extruded in radial direction to form 6 cells),
• 12 for the rhombic dodecahedron,
• 24 for the hexahedron-based surface refined once in the azimuthal directions but not in the radial direction,
• 48 for the rhombic dodecahedron refined once in the azimuthal directions but not in the radial direction,
• 96 for the rhombic dodecahedron refined once. This choice dates from an older version of deal.II before the Manifold classes were implemented: today this choce is equivalent to the rhombic dodecahedron after performing one global refinement.
• Numbers of the kind \(192\times 2^m\) with \(m\geq 0\) integer. This choice is similar to the 24 and 48 cell cases, but provides additional refinements in azimuthal direction combined with a single layer in radial direction. The base mesh is either the 6 or 12 cell version, depending on whether \(m\) in the power is odd or even, respectively.

The versions with 24, 48, and \(2^m 192\) cells are useful if the shell is thin and the radial lengths should be made more similar to the circumferential lengths.

The 3d grids with 12 and 96 cells are plotted below:

Note

This function is declared to exist for triangulations of all space dimensions, but throws an error if called in 1d.

Precondition

The triangulation passed as argument needs to be empty when calling this function.

eccentric_hyper_shell()

template<int dim>

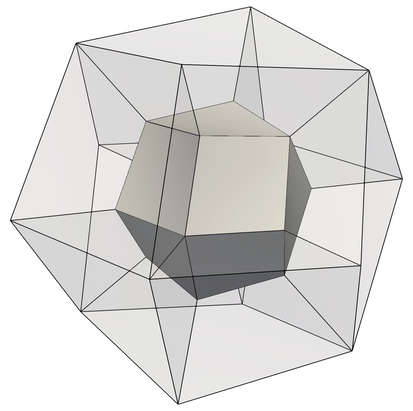
void GridGenerator::eccentric_hyper_shell	(	Triangulation< dim > &	triangulation,
		const Point< dim > &	inner_center,
		const Point< dim > &	outer_center,
		const double	inner_radius,
		const double	outer_radius,
		const unsigned int	n_cells
	)

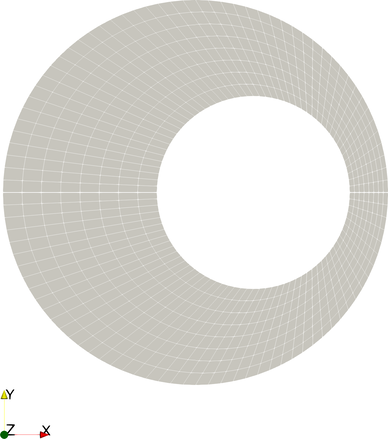
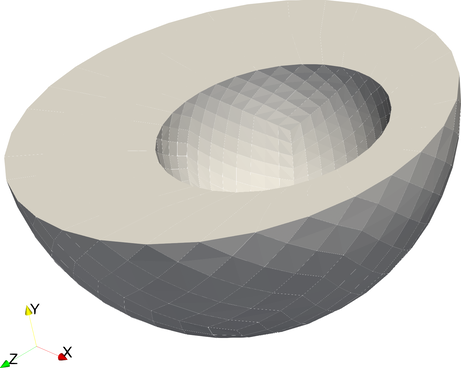
Produce an eccentric hyper-shell, the region between two spheres centered on two distinct center points. One has to specify the inner_center and outer_center, with given inner_radius and outer_radius. The number n_cells indicates the number of cells of the resulting triangulation, i.e., how many cells form the ring (in 2d) or the shell (in 3d).

By default, the outer boundary has the indicator 1 while the inner boundary has id 0. In 3d, this applies to both the faces and the edges of these boundaries.

A SphericalManifold is attached to the outer boundary with an id of 1 while another SphericalManifold is attached to the inner boundary with an id of 0. A TransfiniteInterpolationManifold is attached to all other cells and faces of the triangulation with an id of 2.

Here, the number n_cells of elements has the same meaning as in GridGenerator::hyper_shell.

The grids with a 30% offset of the inner shell in the x direction, 12 initial cells and 3 levels of global refinement are plotted below:

Note

Because it uses the definition of the hyper shell, this function is declared to exist for triangulations of all space dimensions, but throws an error if called in 1d.

Precondition

The triangulation passed as argument needs to be empty when calling this function.

half_hyper_shell()

template<int dim>

void GridGenerator::half_hyper_shell	(	Triangulation< dim > &	tria,
		const Point< dim > &	center,
		const double	inner_radius,
		const double	outer_radius,
		const unsigned int	n_cells = 0,
		const bool	colorize = false
	)

Produce a half hyper-shell, i.e. the space between two circles in two space dimensions and the region between two spheres in 3d, with given inner and outer radius and a given number of elements for this initial triangulation. However, opposed to the previous function, it does not produce a whole shell, but only one half of it, namely that part for which the first component is restricted to non-negative values. The purpose of this class is to enable computations for solutions which have rotational symmetry, in which case the half shell in 2d represents a shell in 3d.

If the number of initial cells n_cells is zero in 2d (as is the default), then it is computed adaptively such that the resulting elements have the least aspect ratio. The argument is ignored in 3d, where the coarse mesh always has 5 cells.

If colorize is set to true, the inner, outer, and the part of the boundary where \(x=0\), get indicator 0, 1, and 2, respectively. Additionally, in 2d, the boundary indicator 3 is given to the vertical edge below the x-axis. Otherwise, if colorize is set to false all indicators are set to 0 (see the glossary entry on colorization).

All manifold ids are set to zero, and a SphericalManifold is attached to the triangulation.

Precondition

The triangulation passed as argument needs to be empty when calling this function.

quarter_hyper_shell()

template<int dim>

void GridGenerator::quarter_hyper_shell	(	Triangulation< dim > &	tria,
		const Point< dim > &	center,
		const double	inner_radius,
		const double	outer_radius,
		const unsigned int	n_cells = 0,
		const bool	colorize = false
	)

Produce a domain that is the intersection between a hyper-shell with given inner and outer radius, i.e. the space between two circles in two space dimensions and the region between two spheres in 3d, and the positive quadrant (in 2d) or octant (in 3d). In 2d, this is indeed a quarter of the full annulus, while the function is a misnomer in 3d because there the domain is not a quarter but one eighth of the full shell.

If the number of initial cells is zero (as is the default), then it is computed adaptively such that the resulting elements have the least aspect ratio in 2d.

If colorize is set to true, the inner, outer, left, and right boundary get indicator 0, 1, 2, and 3 in 2d, respectively. Otherwise all indicators are set to 0. In 3d indicator 2 is at the face \(x=0\), 3 at \(y=0\), 4 at \(z=0\) (see the glossary entry on colorization).

All manifold ids are set to zero, and a SphericalManifold is attached to the triangulation.

Precondition

The triangulation passed as argument needs to be empty when calling this function.

cylinder_shell()

template<int dim>

void GridGenerator::cylinder_shell	(	Triangulation< dim > &	tria,
		const double	length,
		const double	inner_radius,
		const double	outer_radius,
		const unsigned int	n_radial_cells = 0,
		const unsigned int	n_axial_cells = 0
	)

Produce a domain that is the space between two cylinders in 3d, with given length, inner and outer radius and a given number of elements. The cylinder shell is built around the \(z\)-axis with the two faces located at \(z = 0\) and \(z = \) length.

If n_radial_cells is zero (as is the default), then it is computed adaptively such that the resulting elements have the least aspect ratio. The same holds for n_axial_cells.

Note

Although this function is declared as a template, it does not make sense in 1D and 2D. Also keep in mind that this object is rotated and positioned differently than the one created by cylinder().

All manifold ids are set to zero, and a CylindricalManifold is attached to the triangulation.

Precondition

The triangulation passed as argument needs to be empty when calling this function.

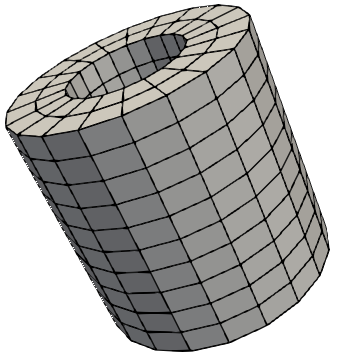
In this picture, a cylinder shell of length 2, inner radius 0.5, outer radius 1 is shown. The default argument for n_radial_cells and n_axial_cells are used and a single global refinement is carried out.

torus()

template<int dim, int spacedim>

void GridGenerator::torus	(	Triangulation< dim, spacedim > &	tria,
		const double	R,
		const double	r,
		const unsigned int	n_cells_toroidal = 6,
		const double	phi = 2.0 *numbers::PI
	)

Produce the volume or surface mesh of a torus. The axis of the torus is the \(y\)-axis while the plane of the torus is the \(x\)- \(z\) plane.

If dim is 3, the mesh will be the volume of the torus, using a mesh equivalent to the circle in the poloidal coordinates with 5 cells on the cross section. This function attaches a TorusManifold to all boundary faces which are marked with a manifold id of 1, a CylindricalManifold to the interior cells and all their faces which are marked with a manifold id of 2 (representing a flat state within the poloidal coordinates), and a TransfiniteInterpolationManifold to the cells between the TorusManifold on the surface and the ToroidalManifold in the center, with cells marked with manifold id 0.

An example for the case if dim is 3 with a cut through the domain at \(z=0\), 6 toroidal cells, \(R=2\) and \(r=0.5\) without any global refinement is given here:

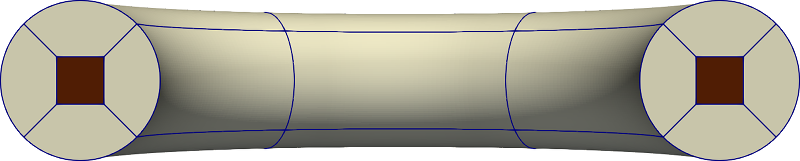
In this picture, the light gray shade represents the manifold id 0 of the transfinite interpolation, which is applied to smoothly add new points between the toroidal shape on the domain boundary and the inner rim where a cylindrical description around the y-axis is prescribed. The inner rim with the manifold id 2 is shown in red shade.

If dim is 2, the mesh will describe the surface of the torus and this function attaches a TorusManifold to all cells and faces (which are marked with a manifold id of 0).

Parameters

tria	The triangulation to be filled.
R	The radius of the circle, which forms the middle line of the torus containing the loop of cells. Must be greater than r.
r	The inner radius of the torus.
n_cells_toroidal	Optional argument to set the number of cell layers in toroidal direction. The default is 6 cell layers.
phi	Optional argument to generate an open torus with angle \(0 < \varphi <= 2 \pi\). The default value is \(2 \pi\), in which case a closed torus is generated. If the torus is open, the torus is cut at two planes perpendicular to the torus centerline. The center of these two planes are located at \((x_1, y_1, z_1) = (R, 0, 0)\) and \((x_2, y_2, z_2) = (R \cos(\varphi), 0, R \sin(\varphi))\).

Note

Implemented for Triangulation<2,3> and Triangulation<3,3>.

hyper_cube_with_cylindrical_hole()

template<int dim>

void GridGenerator::hyper_cube_with_cylindrical_hole	(	Triangulation< dim > &	triangulation,
		const double	inner_radius = .25,
		const double	outer_radius = .5,
		const double	L = .5,
		const unsigned int	repetitions = 1,
		const bool	colorize = false
	)

This function produces a square in the xy-plane with a cylindrical hole in the middle. The square and the circle are centered at the origin. In 3d, this geometry is extruded in \(z\) direction to the interval \([0,L]\).

The inner boundary has a manifold id of \(0\) and a boundary id of \(6\). This function attaches a PolarManifold or CylindricalManifold to the interior boundary in 2d and 3d respectively. The other faces have boundary ids of \(0, 1, 2, 3, 4\), or \(5\) given in the standard order of faces in 2d or 3d.

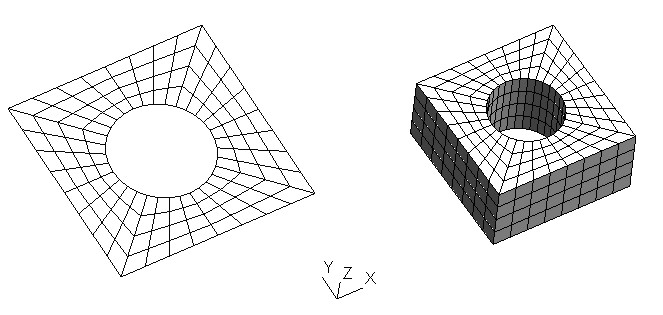
It is implemented in 2d and 3d, and takes the following arguments:

Parameters

triangulation	The triangulation to be filled.
inner_radius	Radius of the internal hole.
outer_radius	Half of the edge length of the square.
L	Extension in z-direction (only used in 3d).
repetitions	Number of subdivisions along the z-direction.
colorize	Whether to assign different boundary indicators to different faces (see the glossary entry on colorization). The colors are given in lexicographic ordering for the flat faces (0 to 3 in 2d, 0 to 5 in 3d) plus the curved hole (4 in 2d, and 6 in 3d). If colorize is set to false, then flat faces get the number 0 and the hole gets number 1.

concentric_hyper_shells()

template<int dim>

void GridGenerator::concentric_hyper_shells	(	Triangulation< dim > &	triangulation,
		const Point< dim > &	center,
		const double	inner_radius = 0.125,
		const double	outer_radius = 0.25,
		const unsigned int	n_shells = 1,
		const double	skewness = 0.1,
		const unsigned int	n_cells_per_shell = 0,
		const bool	colorize = false
	)

Produce a grid consisting of concentric shells. The primary difference between this function and GridGenerator::hyper_shell() is that this function permits unevenly spaced (in the radial direction) coarse level cells.

The parameters center, inner_radius, and outer_radius behave in the same way as the first three arguments to GridGenerator::hyper_shell. n_shells gives the total number of shells to use (i.e., the number of cells in the radial direction). The outer radius of the \(k\)th shell is given by

\[ r = r_{\text{inner}} + (r_\text{outer} - r_\text{inner}) \frac{1 - \tanh(\text{skewness}(1 - k/\text{n\_shells}))} {\tanh(\text{skewness})} \]

where skewness is a parameter controlling the shell spacing in the radial direction: values of skewness close to zero correspond to even spacing, while larger values of skewness (such as \(2\) or \(3\)) correspond to shells biased to the inner radius.

n_cells_per_shell is the same as in GridGenerator::hyper_shell: in 2d the default choice of zero will result in 8 cells per shell (and 12 in 3d). The only valid values in 3d are 6 (the default), 12, and 96 cells: see the documentation of GridGenerator::hyper_shell for more information.

If colorize is true then the outer boundary of the merged shells has a boundary id of \(1\) and the inner boundary has a boundary id of \(0\).

Example: The following code (see, e.g., step-10 for instructions on how to visualize GNUPLOT output)

#include <deal.II/fe/mapping_q_generic.h>
 
#include <deal.II/grid/grid_generator.h>
#include <deal.II/grid/grid_out.h>
#include <deal.II/grid/tria.h>
 
#include <fstream>
 
int main()
{
  using namespace dealii;
 
  Triangulation<2> triangulation;
  GridGenerator::concentric_hyper_shells(triangulation,
                                         Point<2>(),
                                         1.0,
                                         2.0,
                                         5u,
                                         2.0);
 
  GridOut grid_out;
  GridOutFlags::Gnuplot gnuplot_flags(false, 10, true);
  grid_out.set_flags(gnuplot_flags);
 
  const MappingQGeneric<2> mapping(3);
  std::ofstream out("out.gpl");
  grid_out.write_gnuplot(triangulation, out, &mapping);
}

generates the following output:

moebius()

void GridGenerator::moebius	(	Triangulation< 3, 3 > &	tria,
		const unsigned int	n_cells,
		const unsigned int	n_rotations,
		const double	R,
		const double	r
	)

Produce a ring of cells in 3d that is cut open, twisted and glued together again. This results in a kind of moebius-loop.

Parameters

tria	The triangulation to be worked on.
n_cells	The number of cells in the loop. Must be greater than 4.
n_rotations	The number of rotations ( \(\pi/2\) each) to be performed before gluing the loop together.
R	The radius of the circle, which forms the middle line of the torus containing the loop of cells. Must be greater than r.
r	The radius of the cylinder bent together as a loop.

generate_from_name_and_arguments()

template<int dim, int spacedim>

void GridGenerator::generate_from_name_and_arguments	(	Triangulation< dim, spacedim > &	tria,
		const std::string &	grid_generator_function_name,
		const std::string &	grid_generator_function_arguments
	)

Call one of the other GridGenerator functions, parsing the name of the function to call from the string grid_generator_function_name, and the arguments to the function from the string grid_generator_function_arguments.

The string that supplies the arguments is passed to the function Patterns::Tools::Convert<TupleTyple>::to_value(), where TupleType here is a tuple containing all the arguments of the GridGenerator function, including all optional arguments.

An example usage of this function is given by:

GridGenerator::generate_from_name_and_arguments(
  tria,
  "hyper_ball",
  "0.0, 0.0 : 1 : false");

Here, the colon separates the function arguments, and the comma separates the coordinates of a Point<2> argument.

According to the arity of the TupleType, the arguments of the function may be separated by different separators (see the documentation of Patterns::Tuple for the details of how the conversion is performed). If a wrong format is used, an exception is thrown, and the expected format is output as an error message.

All GridGenerator functions are supported. If you find some that are missing, please open an issue on GitHub.

Parameters

tria	The triangulation to be worked on
grid_generator_function_name	The name of the function to call
grid_generator_function_arguments	The arguments of the function, in the format of a tuple-convertible string

Author

Luca Heltai, 2019.

Definition at line 314 of file grid_generator_from_name.cc.

merge_triangulations() [1/2]

template<int dim, int spacedim>

void GridGenerator::merge_triangulations	(	const Triangulation< dim, spacedim > &	triangulation_1,
		const Triangulation< dim, spacedim > &	triangulation_2,
		Triangulation< dim, spacedim > &	result,
		const double	duplicated_vertex_tolerance = 1.0e-12,
		const bool	copy_manifold_ids = false
	)

Given the two triangulations specified as the first two arguments, create the triangulation that contains the cells of both triangulation and store it in the third parameter. Previous content of result will be deleted.

This function is most often used to compose meshes for more complicated geometries if the geometry can be composed of simpler parts for which functions exist to generate coarse meshes. For example, the channel mesh used in step-35 could in principle be created using a mesh created by the GridGenerator::hyper_cube_with_cylindrical_hole function and several rectangles, and merging them using the current function. The rectangles will have to be translated to the right for this, a task that can be done using the GridTools::shift function (other tools to transform individual mesh building blocks are GridTools::transform, GridTools::rotate, and GridTools::scale).

Vertices that are less than duplicated_vertex_tolerance apart will be merged together. It is usually necessary to set this value to something that depends on the input triangulations in some way. One reasonable choice is to use the minimum distance between all adjacent vertices of the input mesh divided by some constant:

auto min_line_length = [](const Triangulation<dim> &tria) -> double
{
  double length = std::numeric_limits<double>::max();
  for (const auto &cell : tria.active_cell_iterators())
    for (unsigned int n = 0; n < GeometryInfo<dim>::lines_per_cell; ++n)
      length = std::min(length, (cell->line(n)->vertex(0) -
                                 cell->line(n)->vertex(1)).norm());
  return length;
};
 
const double tolerance = std::min(min_line_length(triangulation_1),
                                  min_line_length(triangulation_2)) / 2.0;

This will merge any vertices that are closer than any pair of vertices on the input meshes.

Note

The two input triangulations must be coarse meshes, i.e., they can not have any refined cells.

The function copies the material ids of the cells of the two input triangulations into the output triangulation. If copy_manifold_ids is set to true, manifold ids will be copied. Boundary indicators are never copied. In other words, if the two coarse meshes have anything but the default boundary indicators, then you will have to set boundary indicators again by hand in the output triangulation.

This function does not attach any manifolds to result, nor does it set any manifold ids. In particular, manifolds attached to the two input triangulations will be lost in the result triangulation.

For a related operation on refined meshes when both meshes are derived from the same coarse mesh, see GridGenerator::create_union_triangulation().

merge_triangulations() [2/2]

template<int dim, int spacedim>

void GridGenerator::merge_triangulations	(	const std::vector< const Triangulation< dim, spacedim > * > &	triangulations,
		Triangulation< dim, spacedim > &	result,
		const double	duplicated_vertex_tolerance = 1.0e-12,
		const bool	copy_manifold_ids = false
	)

Same as above but allows to merge more than two triangulations at once. The following gives an example of how to use this function:

Triangulation<2> tria_1, tria_2, tria_3;
// initialize tria_1, tria_2 and tria_3
...
Triangulation<2> merged_triangulation;
GridGenerator::merge_triangulations({&tria_1, &tria_2, &tria_3},
                                    merged_triangulation,
                                    1.0e-10,
                                    false);

replicate_triangulation()

template<int dim, int spacedim = dim>

void GridGenerator::replicate_triangulation	(	const Triangulation< dim, spacedim > &	input,
		const std::vector< unsigned int > &	extents,
		Triangulation< dim, spacedim > &	result
	)

Replicate a given triangulation in multiple coordinate axes.

Parameters

input	The triangulation which will be replicated along the coordinate axes.
extents	A vector with dim entries specifying how many copies of a triangulation should be present along each coordinate axis.
result	The triangulation to be created. It needs to be empty upon calling this function.

This function creates a new Triangulation equal to a dim-dimensional array of copies of input. Copies of input are created by translating input along the coordinate axes. Boundary ids of faces (but not lines in 3D) and all manifold ids are copied but Manifold objects are not since most Manifold objects do not work correctly when a Triangulation has been translated.

To see how this works, consider the following code:

Triangulation<2> input;
GridGenerator::hyper_cube_with_cylindrical_hole(input);
Triangulation<2> output;
GridGenerator::replicate_triangulation(input, {3, 2}, output);

results in

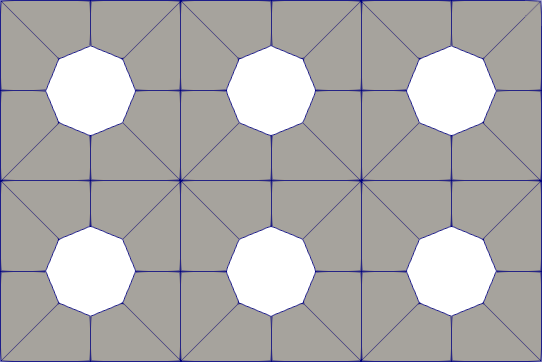
And, similarly, in 3D:

Triangulation<3> input;
GridGenerator::hyper_cross(1, 1, 1, 2, 1, 2);
Triangulation<3> output;
GridGenerator::replicate_triangulation(input, {3, 2, 1}, output);

results in

Note

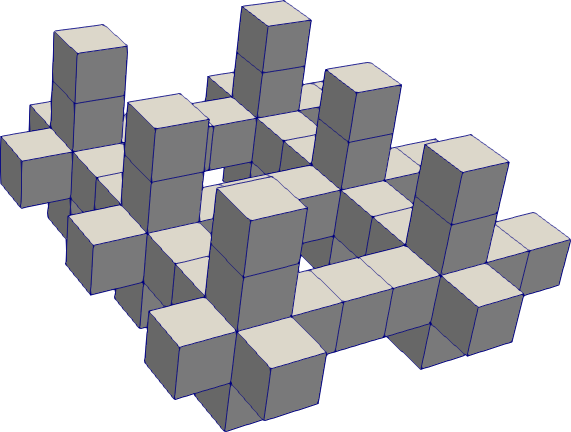
This function determines the spacing of the copies of input based on the BoundingBox of input. If the boundary faces of input are not aligned with the coordinate axes then the copies might not share common faces; i.e., this function is intended for simple geometries with boundary faces aligned along the coordinate axes.

create_union_triangulation()

template<int dim, int spacedim>

void GridGenerator::create_union_triangulation	(	const Triangulation< dim, spacedim > &	triangulation_1,
		const Triangulation< dim, spacedim > &	triangulation_2,
		Triangulation< dim, spacedim > &	result
	)

Given the two triangulations specified as the first two arguments, create the triangulation that contains the finest cells of both triangulation and store it in the third parameter. Previous content of result will be deleted.

Note

This function is intended to create an adaptively refined triangulation that contains the most refined cells from two input triangulations that were derived from the same coarse mesh by adaptive refinement. This is an operation sometimes needed when one solves for two variables of a coupled problem on separately refined meshes on the same domain (for example because these variables have boundary layers in different places) but then needs to compute something that involves both variables or wants to output the result into a single file. In both cases, in order not to lose information, the two solutions can not be interpolated onto the respectively other mesh because that may be coarser than the ones on which the variable was computed. Rather, one needs to have a mesh for the domain that is at least as fine as each of the two initial meshes. This function computes such a mesh.

If you want to create a mesh that is the merger of two other coarse meshes, for example in order to compose a mesh for a complicated geometry from meshes for simpler geometries, then this is not the function for you. Instead, consider GridGenerator::merge_triangulations().

This function assumes that both triangulation_1 and triangulation_2 have the same manifold descriptions. The output Triangulation has the same manifold ids as these two triangulations.

Both of the source conditions need to be available entirely locally. In other words, they can not be objects of type parallel::distributed::Triangulation.

create_triangulation_with_removed_cells()

template<int dim, int spacedim>

void GridGenerator::create_triangulation_with_removed_cells	(	const Triangulation< dim, spacedim > &	input_triangulation,
		const std::set< typename Triangulation< dim, spacedim >::active_cell_iterator > &	cells_to_remove,
		Triangulation< dim, spacedim > &	result
	)

This function creates a triangulation that consists of the same cells as are present in the first argument, except those cells that are listed in the second argument. The purpose of the function is to generate geometries subtractively from the geometry described by an existing triangulation. A prototypical case is a 2d domain with rectangular holes. This can be achieved by first meshing the entire domain and then using this function to get rid of the cells that are located at the holes. Likewise, you could create the mesh that GridGenerator::hyper_L() produces by starting with a GridGenerator::hyper_cube(), refining it once, and then calling the current function with a single cell in the second argument.

Parameters

[in]	input_triangulation	The original triangulation that serves as the template from which the new one is to be created.
[in]	cells_to_remove	A list of cells of the triangulation provided as first argument that should be removed (i.e., that should not show up in the result.
[out]	result	The resulting triangulation that consists of the same cells as are in input_triangulation, with the exception of the cells listed in cells_to_remove.

Note

Unlike most GridGenerator functions, this function does not attach any manifolds to result, nor does it set any manifold ids.

Precondition

Because we cannot create triangulations de novo that contain adaptively refined cells, the input triangulation needs to have all of its cells on the same level. Oftentimes, this will in fact be the coarsest level, but it is allowed to pass in a triangulation that has been refined globally a number of times. The output triangulation will in that case simply be a mesh with only one level that consists of the active cells of the input minus the ones listed in the second argument. However, the input triangulation must not have been adaptively refined.

extrude_triangulation() [1/4]

void GridGenerator::extrude_triangulation	(	const Triangulation< 2, 2 > &	input,
		const unsigned int	n_slices,
		const double	height,
		Triangulation< 3, 3 > &	result,
		const bool	copy_manifold_ids = false,
		const std::vector< types::manifold_id > &	manifold_priorities = {}
	)

Extrude input in the \(z\) direction from \(z = 0\) to \(z = \text{height}\). The number of slices, or layers of cells perpendicular to the \(z = 0\) plane, will be n_slices slices (minimum is 2). The boundary indicators of the faces of input will be assigned to the corresponding side walls in \(z\) direction. The bottom and top get the next two free boundary indicators: i.e., if input has boundary ids of \(0\), \(1\), and \(42\), then the \(z = 0\) boundary id of result will be \(43\) and the \(z = \text{height}\) boundary id will be \(44\).

This function does not, by default, copy manifold ids. The reason for this is that there is no way to set the manifold ids on the lines of the resulting Triangulation without more information: for example, if two faces of input with different manifold ids meet at a shared vertex then there is no a priori reason to pick one manifold id or another for the lines created in result that are parallel to the \(z\)-axis and pass through that point. If copy_manifold_ids is true then this function sets line manifold ids by picking the one that appears first in manifold_priorities. For example: if manifold_priorities is {0, 42, numbers::flat_manifold_id} and the line under consideration is adjacent to faces with manifold ids of 0 and 42, then that line will have a manifold id of 0. The correct ordering is almost always

1. manifold ids set on the boundary,
2. manifold ids that describe most of the cells in the Triangulation (e.g., numbers::flat_manifold_id), and
3. any manifold ids corresponding to TransfiniteInterpolationManifold manifolds.

In particular, since TransfiniteInterpolationManifold interpolates between surrounding manifolds, its manifold id should usually not be set on lines or faces that are adjacent to cells with different manifold ids. The default value for manifold_priorities follows this ranking (where each category is sorted in ascending order):

1. manifold ids associated with manifolds that are not TransfiniteInterpolationManifold, and
2. manifold ids associated with any TransfiniteInterpolationManifold objects.

Note that numbers::flat_manifold_id (should it be a manifold id of input) will always be the last entry in the first category.

Precondition

The 2d input triangulation input must be a coarse mesh, i.e., it cannot have any refined cells.

Note

Since input and output have different spatial dimensions, no manifold objects are copied by this function regardless of the value of copy_manifold_ids.

extrude_triangulation() [2/4]

void GridGenerator::extrude_triangulation	(	const Triangulation< 2, 2 > &	input,
		const unsigned int	n_slices,
		const double	height,
		Triangulation< 2, 2 > &	result,
		const bool	copy_manifold_ids = false,
		const std::vector< types::manifold_id > &	manifold_priorities = {}
	)

Overload of extrude_triangulation() to allow dimension independent code to compile. This function throws an error when called, as extrude_triangulation() is only implemented to extrude a dim=2 to a dim=3 Triangulation.

extrude_triangulation() [3/4]

void GridGenerator::extrude_triangulation	(	const Triangulation< 2, 2 > &	input,
		const std::vector< double > &	slice_coordinates,
		Triangulation< 3, 3 > &	result,
		const bool	copy_manifold_ids = false,
		const std::vector< types::manifold_id > &	manifold_priorities = {}
	)

Overload of the previous function. Take a 2d Triangulation that is being extruded. Differing from the previous function taking height and number of slices for uniform extrusion, this function takes z-axis values slice_coordinates where the slicing will happen. The boundary indicators of the faces of input are going to be assigned to the corresponding side walls in z direction. The bottom and top get the next two free boundary indicators.

Precondition

The 2d input triangulation input must be a coarse mesh, i.e., it cannot have any refined cells.

Note

Since input and output have different spatial dimensions no manifold objects are copied (nor are any manifold ids set) by this function.

Author

Weixiong Zheng, 2018

extrude_triangulation() [4/4]

void GridGenerator::extrude_triangulation	(	const Triangulation< 2, 2 > &	input,
		const std::vector< double > &	slice_coordinates,
		Triangulation< 2, 2 > &	result,
		const bool	copy_manifold_ids = false,
		const std::vector< types::manifold_id > &	manifold_priorities = {}
	)

Overload of extrude_triangulation() to allow dimension independent code to compile. This function throws an error when called, as extrude_triangulation() is only implemented to extrude a dim=2 to a dim=3 Triangulation.

flatten_triangulation()

template<int dim, int spacedim1, int spacedim2>

void GridGenerator::flatten_triangulation	(	const Triangulation< dim, spacedim1 > &	in_tria,
		Triangulation< dim, spacedim2 > &	out_tria
	)

Given an input triangulation in_tria, this function makes a new flat triangulation out_tria which contains a single level with all active cells of the input triangulation. If spacedim1 and spacedim2 are different, only the smallest spacedim components of the vertices are copied over. This is useful to create a Triangulation<2,3> out of a Triangulation<2,2>, or to project a Triangulation<2,3> into a Triangulation<2,2>, by neglecting the z components of the vertices.

No internal checks are performed on the vertices, which are assumed to make sense topologically in the target spacedim2 dimensional space. If this is not the case, you will encounter problems when using the triangulation later on.

All information about cell manifold_ids and material ids are copied from one triangulation to the other, and only the boundary manifold_ids and boundary_ids are copied over from the faces of in_tria to the faces of out_tria. If you need to specify manifold ids on interior faces, they have to be specified manually after the triangulation is created.

This function will fail if the input Triangulation is of type parallel::distributed::Triangulation, as well as when the input Triangulation contains hanging nodes.

Parameters

[in]	in_tria	The base input for a new flat triangulation.
[out]	out_tria	The desired flattened triangulation constructed from the in_tria.

Note

Since input and output have different spatial dimensions no manifold objects are copied by this function: you must attach new manifold objects to out_tria.

Author

Luca Heltai, 2014

extract_boundary_mesh()

template<template< int, int > class MeshType, int dim, int spacedim>

std::map<typename MeshType<dim - 1, spacedim>::cell_iterator, typename MeshType<dim, spacedim>::face_iterator> GridGenerator::extract_boundary_mesh	(	const MeshType< dim, spacedim > &	volume_mesh,
		MeshType< dim - 1, spacedim > &	surface_mesh,
		const std::set< types::boundary_id > &	boundary_ids = std::set< types::boundary_id >()
	)

This function implements a boundary subgrid extraction. Given a <dim,spacedim>-Triangulation (the "volume mesh") the function extracts a subset of its boundary (the "surface mesh"). The boundary to be extracted is specified by a list of boundary_ids. If none is specified the whole boundary will be extracted. The function is used in step-38.

The function also builds a mapping linking the cells on the surface mesh to the corresponding faces on the volume one. This mapping is the return value of the function.

Note

The function builds the surface mesh by creating a coarse mesh from the selected faces of the coarse cells of the volume mesh. It copies the boundary indicators of these faces to the cells of the coarse surface mesh. The surface mesh is then refined in the same way as the faces of the volume mesh are. In order to ensure that the surface mesh has the same vertices as the volume mesh, it is therefore important that you assign appropriate boundary descriptions through Triangulation::set_manifold() to the surface mesh object before calling this function. If you don't, the refinement will happen under the assumption that all faces are straight (i.e using the FlatManifold class) rather than utilizing the Manifold object you may want to use to determine the location of new vertices.

Template Parameters

MeshType	A type that satisfies the requirements of the MeshType concept. The map that is returned will be between cell iterators pointing into the container describing the surface mesh and face iterators of the volume mesh container. If MeshType is DoFHandler or hp::DoFHandler, then the function will re-build the triangulation underlying the second argument and return a map between appropriate iterators into the MeshType arguments. However, the function will not actually distribute degrees of freedom on this newly created surface mesh.
dim	The dimension of the cells of the volume mesh. For example, if dim==2, then the cells are quadrilaterals that either live in the plane, or form a surface in a higher-dimensional space. The dimension of the cells of the surface mesh is consequently dim-1.
spacedim	The dimension of the space in which both the volume and the surface mesh live.

Parameters

[in]	volume_mesh	A container of cells that define the volume mesh.
[out]	surface_mesh	A container whose associated triangulation will be built to consist of the cells that correspond to the (selected portion of) the boundary of the volume mesh.
[in]	boundary_ids	A list of boundary indicators denoting that subset of faces of volume cells for which this function should extract the surface mesh. If left at its default, i.e., if the set is empty, then the function operates on all boundary faces.

Returns

A map that for each cell of the surface mesh (key) returns an iterator to the corresponding face of a cell of the volume mesh (value). The keys include both active and non-active cells of the surface mesh. The order of vertices of surface cells and the corresponding volume faces may not match in order to ensure that each surface cell is associated with an outward facing normal. As a consequence, if you want to match quantities on the faces of the domain cells and on the cells of the surface mesh, you may have to translate between vertex locations or quadrature points.

Note

The algorithm outlined above assumes that all faces on higher refinement levels always have exactly the same boundary indicator as their parent face. Consequently, we can start with coarse level faces and build the surface mesh based on that. It would not be very difficult to extend the function to also copy boundary indicators from finer level faces to their corresponding surface mesh cells, for example to accommodate different geometry descriptions in the case of curved boundaries (but this is not currently implemented).

Since volume_mesh and surface_mesh have different spatial dimensions no manifold objects are copied by this function: you must attach new manifold objects to surface_mesh.